Calcea Johnson and Ne’kiya Jackson, teen students at St. Mary’s Academy in New Orleans, announce their remarkable discovery. (Photo: screenshot via WWLTV)
In school, all students are taught the Pythagorean Theorem at some point. It is an ancient formula—named after sixth-century BCE Greek mathematician Pythagoras—which historians believe was independently developed in different ways across the ancient world in Mesopotamia, India, and Egypt. Building on ancient proofs of the theorem, mathematicians over the past two millennia have developed around 350 proofs to show its validity. However, it has long been believed that proving the theorem with trigonometry (the math of angles) was not possible. In an amazing announcement, two teens from New Orleans presented their finding of four such new proofs at a conference of the American Mathematical Society, causing excitement in the mathematical world.
The Pythagorean Theorem can be used to find the length of one side of a right triangle (a triangle with a 90-degree angle): if you have the lengths of the perpendicular sides a and b, for example, you plug the values into the formula a^2+b^2=c^2. Solve for c and you can find the length of the remaining side (the hypotenuse). The Pythagorean Theorem has applications in countless regions of math and engineering. Ancient peoples frequently used Pythagorean triples, a set of three whole numbers which satisfy the equation—for example, 3, 4, and 5. Early proofs for the theorem were geometric, combining the areas of squares to show how the math works.
More recent proofs have gotten creative, for example, by using differentials or area-preserving shearing. Calcea Johnson and Ne'Kiya Jackson, teen students at St. Mary's Academy in New Orleans were also ready to get creative. “In the 2000 years since trigonometry was discovered, it's always been assumed that any alleged proof of Pythagoras's Theorem based on trigonometry must be circular,” they told an audience at the American Mathematical Society Southeastern Regional Conference. “In fact, in the book containing the largest known collection of proofs (The Pythagorean Proposition by Elisha Loomis) the author flatly states that ‘There are no trigonometric proofs, because all the fundamental formulae of trigonometry are themselves based upon the truth of the Pythagorean Theorem.'”
Triumphantly, the teens announced, “But that isn't quite true: in our lecture, we present a new proof of Pythagoras's Theorem which is based on a fundamental result in trigonometry—the Law of Sines—and we show that the proof is independent of the Pythagorean trig identity \sin^2x + \cos^2x = 1.” Reportedly, the watching mathematicians (college and professional level) started to get excited. “I saw a bunch of people writing down stuff and pulling up things on their computers. And they really connected with this,” Johnson said. “We got a lot of congratulations…Some people apparently started recording.” In total, the teens presented four new proofs based on trig, an exciting development for the entire profession.
The teens credited their “great teachers” and their school's ethos of hard work for helping them to keep pushing their mathematical skills. American Mathematical Society Executive Director Catherine Roberts suggested the teens submit their new proofs to a peer-reviewed journal to “determine whether their proof is a correct contribution to the mathematics literature.” STEM needs more young women, especially young women of color. For these two teens, youth is not a barrier to innovation. “It's really an unparalleled feeling, honestly, because there's just nothing like being able to do something that people don't think young people can do,” Johnson told WWLTV. “A lot of times you see this stuff, you don't see kids like us doing it.”
These two teens developed a trigonometry proof for the famous Pythagorean Theorem, something mathematicians have long thought impossible.
The teens presented their proof at an American Mathematical Society conference.
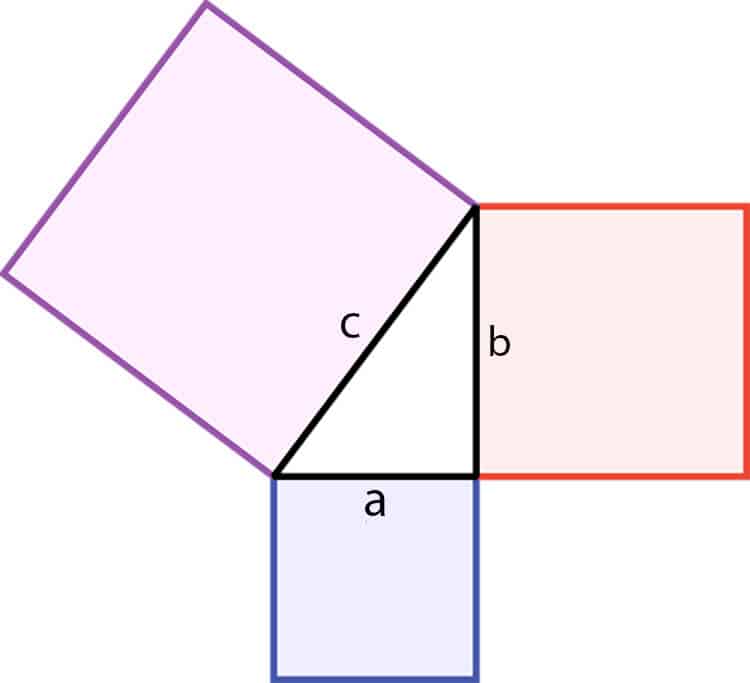
A simple and long-known Pythagorean Theorem proof. (Photo: edited from Wapcaplet's version, Wikimedia Commons, CC BY-SA 3.0)
Participants were excited at the news, and the girls have been encouraged to submit their proof to peer review through a scholarly journal.
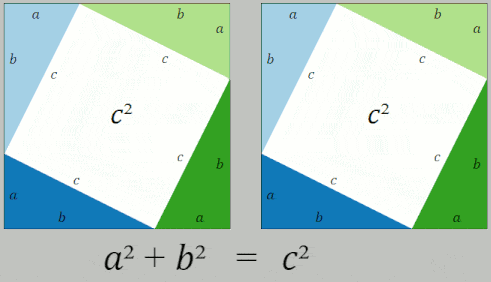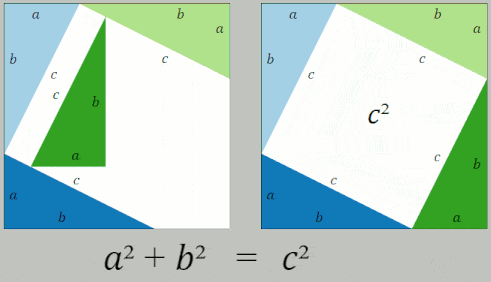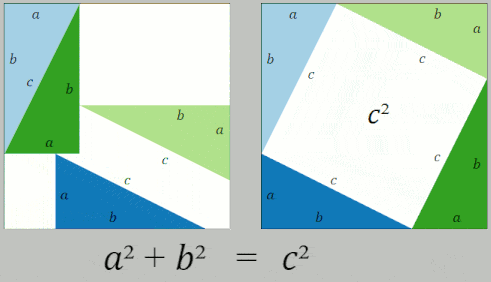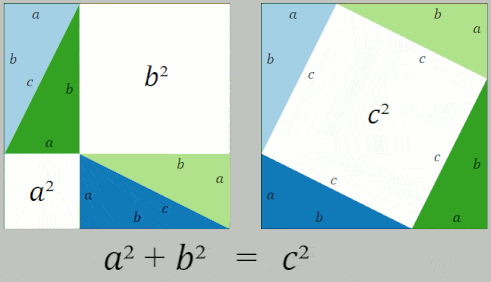
Another simple, well-known proof. (Photo: William B. Faulk via Wikimedia Commons, CC BY-SA 4.0)
Related Articles:
Watch a Mathematician Effortlessly Fit a Large Circular Disk Through a Smaller Square Hole
Artist Combines Passion for Math, Nature, and Art to Create Incredible Topographical Art
25 Creative Gifts For People Who Love Math and Science
Take a Tour of Tessellations, the Mathematical Art of Repeating Patterns